Numerical simulations of the flow about a 6:1 prolate spheroid at an angle of incidence of α = 20o and Reynolds number of Re∞ = 4.2 × 106 were conducted using various Reynolds Stress (RSM) and two-equation turbulence models implemeted in the EZNSS code. These flow conditions correspond to an experiment conducted by Simpson et al. (http://www.dept.aoe.vt.edu/~simpson/prolatespheroid/).
Despite its simple geometry, the flow around a prolate spheroid at incidence exhibits complex flow features, such as cross-flow separation, streamline curvature, the formation and evolution of free-vortex sheets, and stream-wise vortices. For moderate incidence angles, the flow separates from the leeward side of the prolate spheroid and rolls up into coherent longitudinal symmetric vortices. A pair of primary vortices are usually formed, accompanied by at least one pair of secondary vortices.
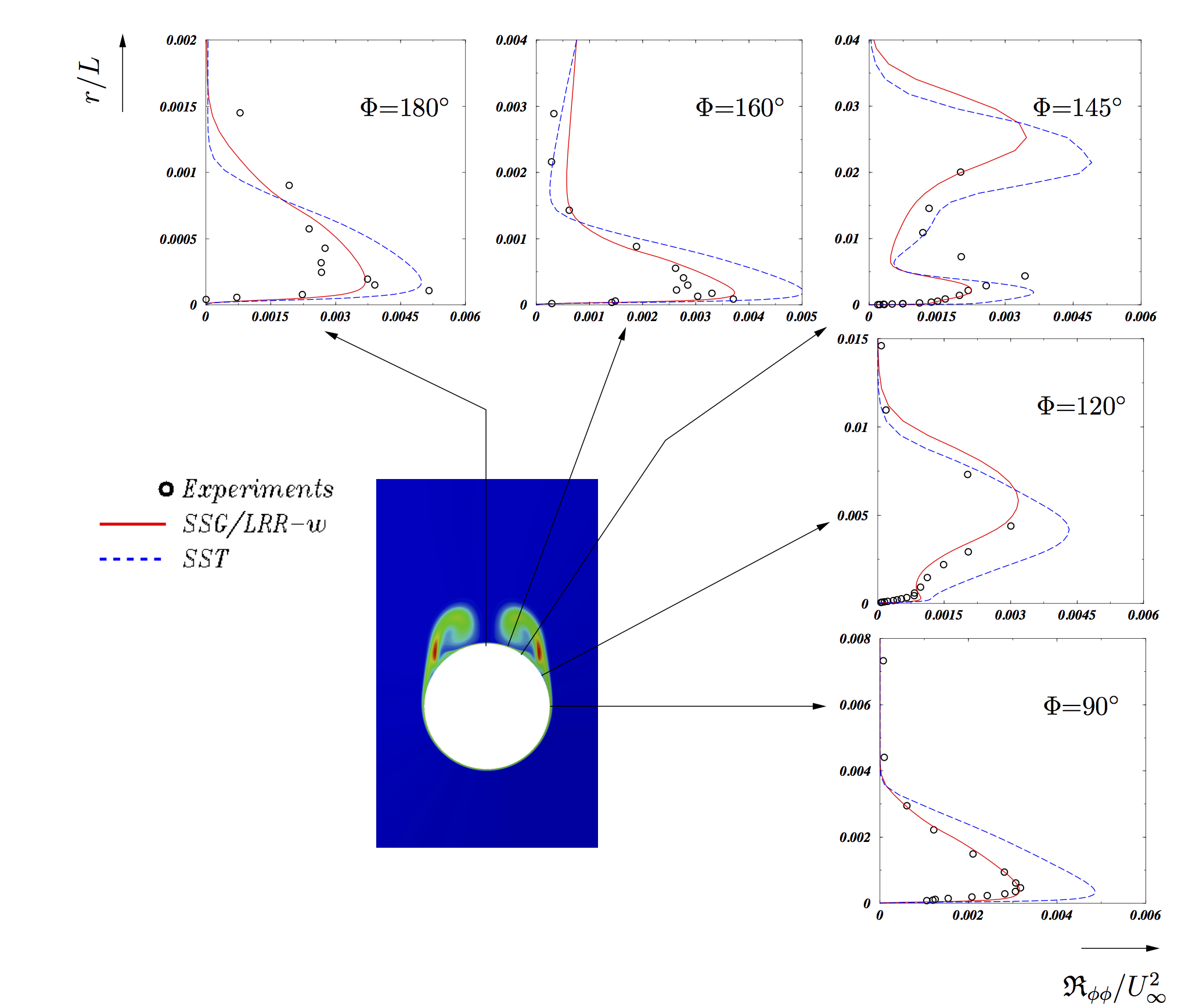
The following figures show a comparison of the computed and experimentally measured boundary layer velocity profiles, and Reynolds stress compnents at five radial rakes along station x/L = 0.772. The computed results are in excellent agreement with experimental data, however some discrepancies may be noted in simulation results obtained with the kω-SST model. Specifically, the very near wall region is by far more accurately predicted using the RSM-ω model. This case strongly demonstrates the superiority of Reynolds stress models in accurate prediction of separated flows, and accentuates the need for RSM model development.
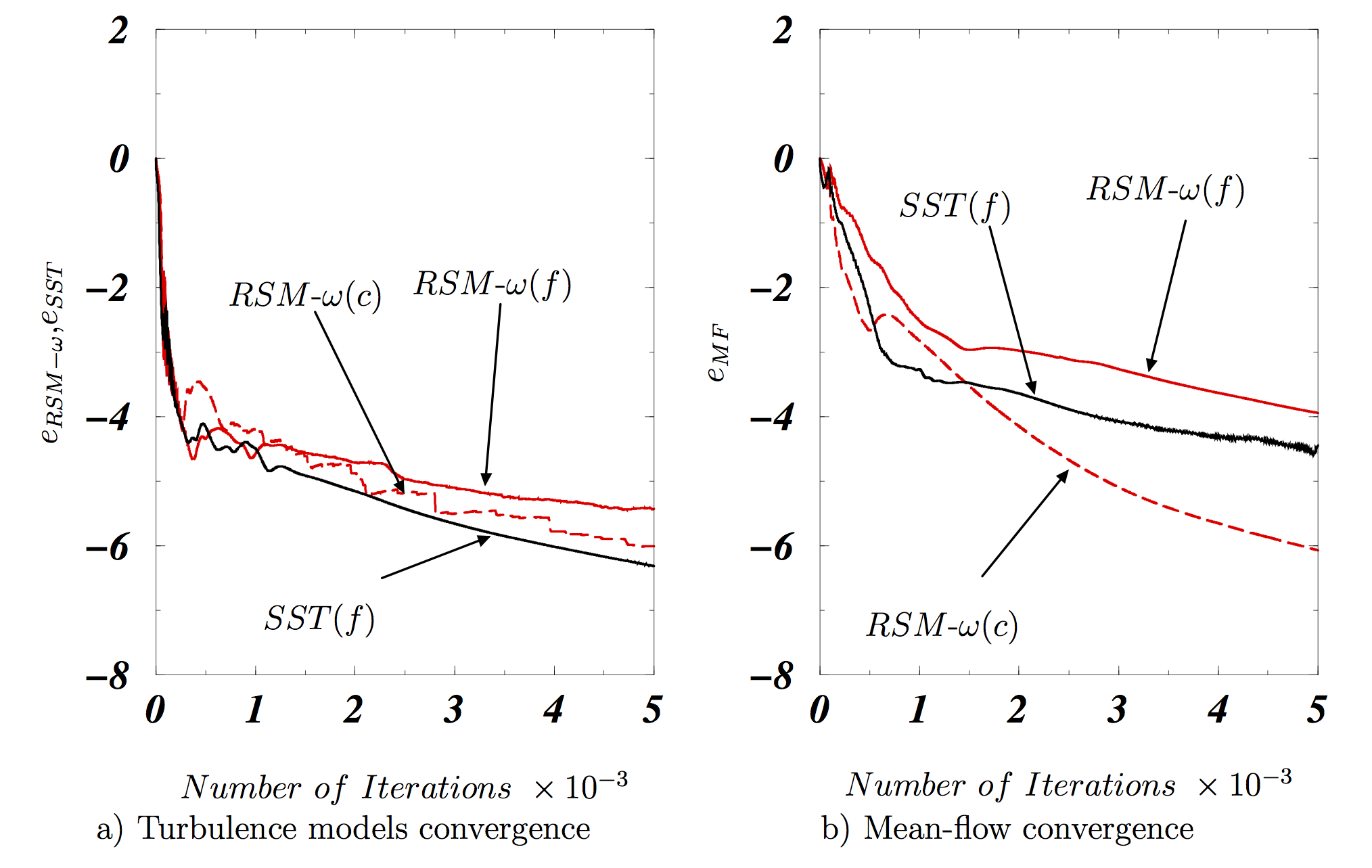
The vortical structure on the lee side presents a serious obstacle for numerical flow predictions. Nevertheless, as may be seen in the following Figure, the special unconditionally positive-convergent (UPC) implicit scheme developed by ISCFDC enables convergence for CFL numbers as high as 40 for the mean-flow equations, and up to 1000000 for the turbulence model equations.